---

BY

MUHAMMET ARICAN

---

Assignment 4 : Centers of a Triangle

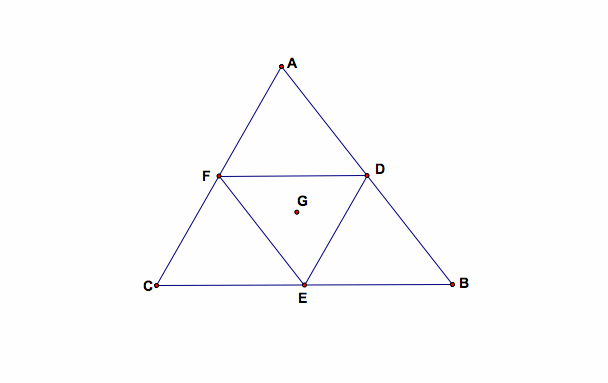
The Centroid of a triangle is the point where all three medians intersect. The Centroid is generally shown with the letter G. A median is a line segment or a ray that connects one of the vertices of the triangle to the midpoint of the opposite side. A median divides the side to the two equal parts such that |BE|=|AE|, |AD|=|CD|, and |BF|=|CF|. Below is a construction that should help you visualize a centroid.

 

G is the CENTROID of ABC triangle.Here ABC is a Acute triangle.

 

There are several important specifics of the Centroid and Medians. Some of these are:

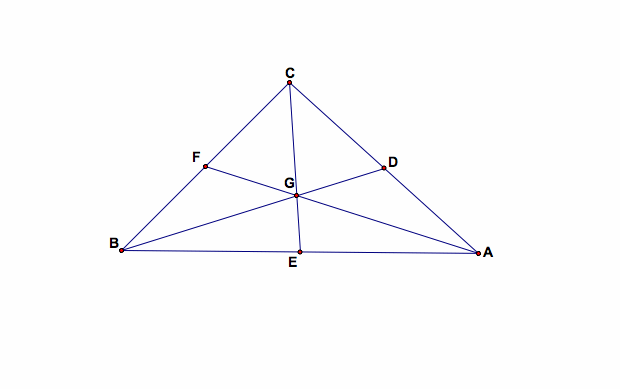
• One of the most important specifics of Medians and the Centroid is that they divide the triangle to the six equal areas such that the area of CDG, DAG, GAE, GEB, FGB, and CGF triangles are equal. One can see this relations from above graph.

 

• The distance between the Centroid and a vertice of the triangle is equal to two times the distance between the Centroid and a midpoint. For example from above graph : |CG|=2|GE|, |AG|=2|GF|, and |BG|=2|GD|

 

• If we connect the Centroid and vertices then we will have three triangles in side the big triangle and these triangles have the same area. For example from below graph : CGB, CAG, and BGA triangles have the same area.

 

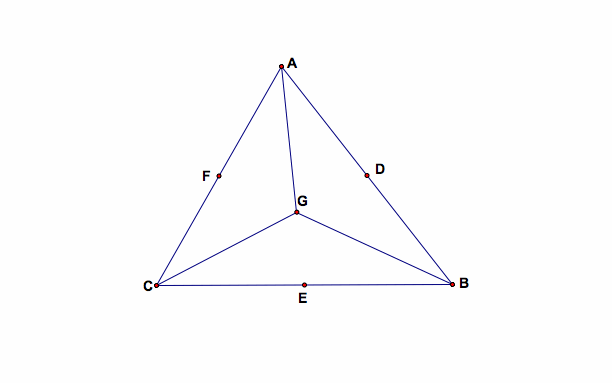
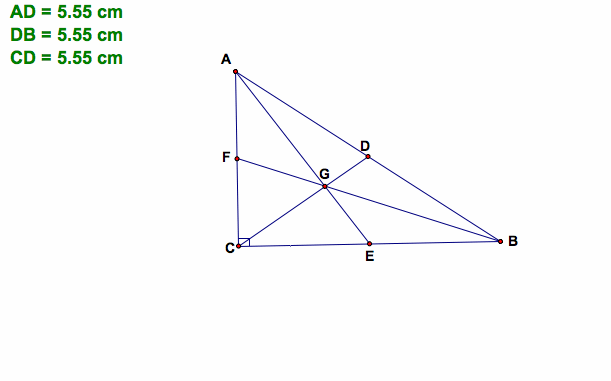
• For a right triangle the distance of Hypotenuse is equal to 2 times the distance of the median which connects the vertices of the triangle to the midpoint of the Hypotenuse. For example from below graph : |AD|=|BD|= |CD| see the right triangle below.

 

Right Triangle

 

• If we connect the Centroid and midpoints then we will have three quadrilaterals and these quadrilaterals going to have the same area such that the area of ADGF, DBEG, and GECF have the same area. One can see this from below graph:

 

 

• If we draw a parallel segment from midpoint F to D and call the intersection point K then |AK|=|KE|.

 

 

• Any median divides the triangle into two equal areas such that the line segment CE divides any given triangle into two congruent areas such that CEB and CAE. A similar situation occurs for all other medians.

 

 

• If we connect midpoints then as one can see from below graph we will have four triangles and these triangles will have the same area.